Fact: Any equation involving fractions and decimals can be turned into an equation without fractions and decimals.
Let's see how!
Example $$ a+\frac{3}{4}=\frac{3}{8}a-\frac{1}{2} $$
Solution
$$ \begin{array}{rll} \displaystyle a+\frac{3}{4}&\displaystyle= \frac{3}{8}a-\frac{1}{2}&\mbox{original equation}\\ \displaystyle \color{magenta}{8 \cdot} \left(a+\frac{3}{4}\right)&\displaystyle= \color{magenta}{8 \cdot}\left(\frac{3}{8}a-\frac{1}{2}\right)&\mbox{multiply both sides by a number which cancels all denoms}\\ \displaystyle \color{magenta}{8 \cdot} a+\color{magenta}{8 \cdot}\frac{3}{4}&\displaystyle= \color{magenta}{8 \cdot}\frac{3}{8}a-\color{magenta}{8 \cdot}\frac{1}{2}&\mbox{distribute}\\ \displaystyle 8a+6&\displaystyle= 3a-4&\mbox{simplify (no denoms!)}\\ \end{array} $$ Now we may proceed as before! $$ \begin{array}{rll} \displaystyle 8a+6\color{magenta}{-3a}&\displaystyle= 3a-4\color{magenta}{-3a}&\mbox{subtraction property}\\ \displaystyle 5a+6&\displaystyle= -4\color{magenta}{-3a}&\mbox{}\\ \displaystyle 5a+6\color{magenta}{-6}&\displaystyle=-4\color{magenta}{-6}&\mbox{subtraction property}\\ \displaystyle 5a&\displaystyle=-10&\mbox{simplify}\\ \displaystyle \frac{5a}{\color{magenta}{5}}&\displaystyle=\frac{-10}{\color{magenta}{5}}&\mbox{division property}\\ \displaystyle a&\displaystyle=-2&\mbox{simplify}\\ \end{array} $$ Check $$ \begin{array}{rll} \displaystyle \color{magenta}{a}+\frac{3}{4}&\displaystyle= \frac{3}{8}\color{magenta}{a}-\frac{1}{2}&\mbox{original equation}\\ \displaystyle \color{magenta}{(-2)}+\frac{3}{4}&\displaystyle= \frac{3}{8}\color{magenta}{(-2)}-\frac{1}{2}&\mbox{since $\displaystyle \color{magenta}{a=-2}$}\\ \displaystyle -\frac{8}{4}+\frac{3}{4}&\displaystyle=-\frac{6}{8}-\frac{1}{2} & \mbox{}\\ \displaystyle -\frac{5}{4}&\displaystyle=-\frac{3}{4}-\frac{2}{4} & \mbox{}\\ \displaystyle -\frac{5}{4}&\displaystyle=-\frac{5}{4} & \mbox{$\checkmark$}\\ \end{array} $$
Notice that we had to do a "prep step" before applying our general strategy for solving equations.
General Strategy for Solving Equations
Step 0: (The "Prep Step"). Clear any fractions or decimals by multiplying each term by a single number which clears each decimal or fraction.
Step 1: Simplify each side of the equation as much as possible.
Step 2: Collect the variable terms to the "variable" side of the equation, using the Addition or Subtraction Property of Equality.
Step 3: Collect all the constants to the "constant" side of the equation, using the Addition or Subtraction Property of Equality.
Step 4: Make the coefficient of the variable equal $1,$ using the Multiplication or Division Property of Equality.
Step 5: Check the solution by substituting it into the original equation.
Examples
(a) $\displaystyle -5=\frac{1}{4}(8x+4)$
(b) $\displaystyle \frac{5x-3}{4}=\frac{x}{2}$
(c) $\displaystyle \frac{4q+3}{2}=\frac{3q+5}{4}$
Solutions
(a) 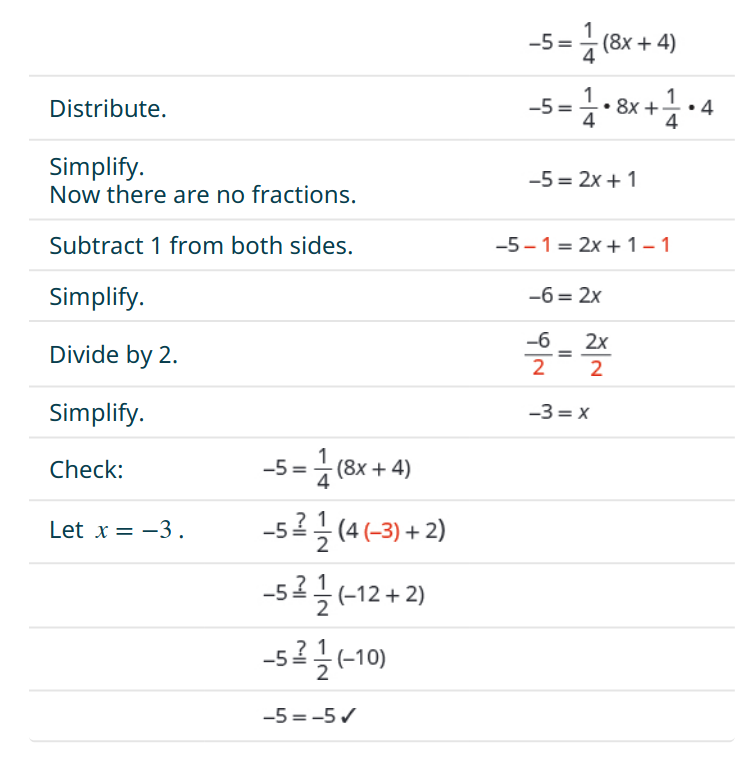

(b) 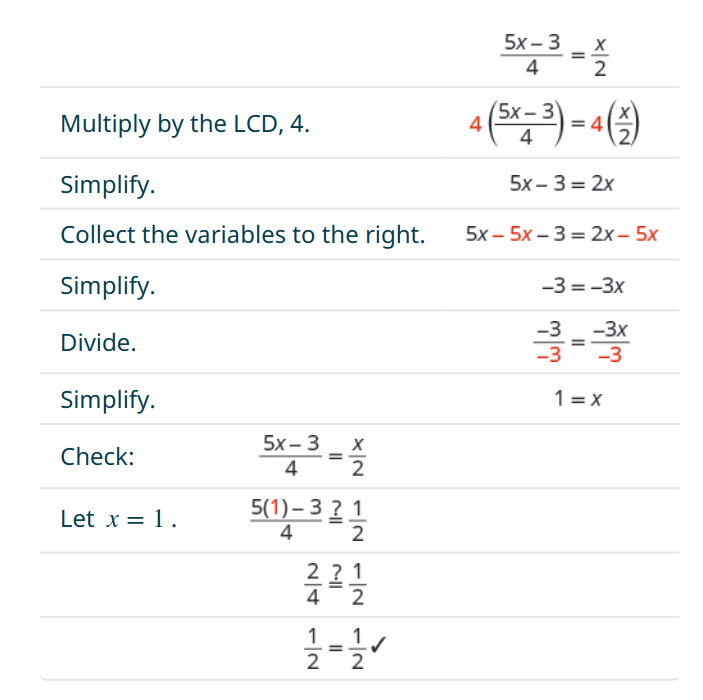

(c) 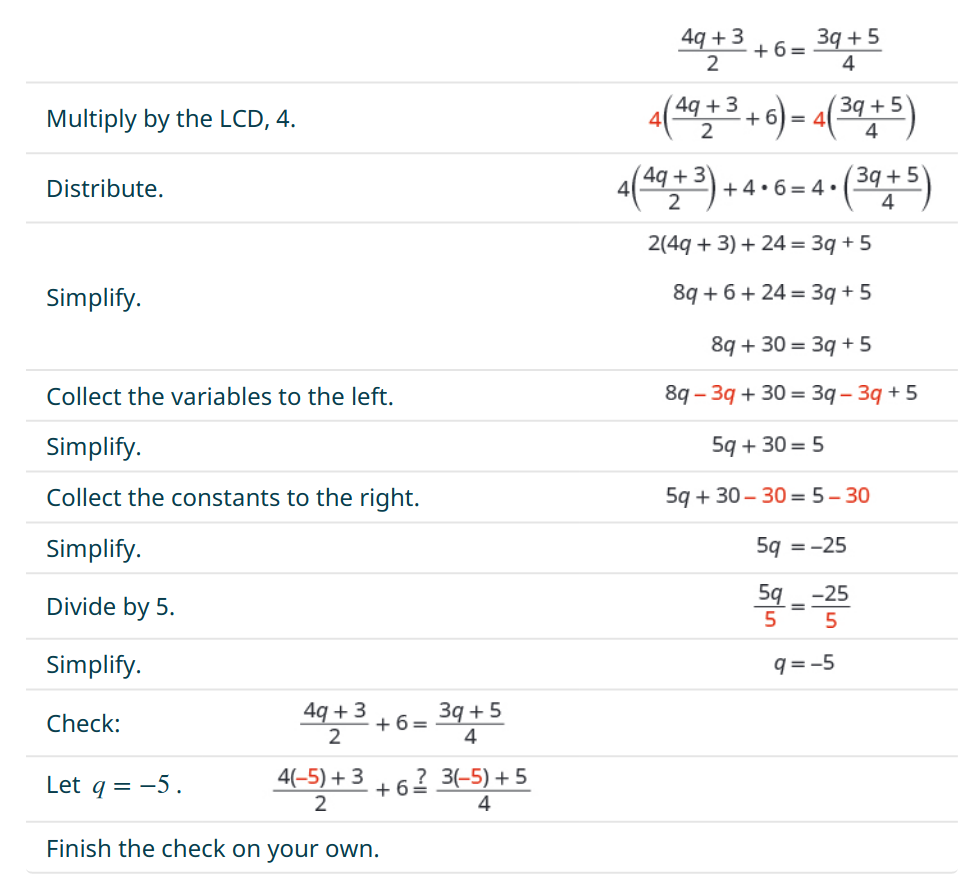

For equations involving decimals, we multiply by the appropriate power of $10.$
That is, $10,$ $100,$ $1000,$ $10000,$ etc.
Let's see it in action!
Examples
$0.25x + 0.05(x + 3) = 2.85$
Solution

Translation Practice
Taylor has $\$2.00$ in dimes and pennies.
The only other thing we know is that Taylor has $2$ more pennies than dimes.
How many pennies and dimes does Taylor have?