Today we are going to learn some shortcuts for dealing with OR outcomes.
That is, we will learn to deal with probabilities that look like $P(A \cup B).$
A Big Idea: Mutually Exclusive Events
Two events are mutually exclusive if they have no outcomes in common.
Example
Suppose you roll a die. The events "roll an even number" and "roll an odd number" are mutually exclusive since these events have no outcomes in common.
$\{$
 $,$
$,$
 $,$
$,$
 $\}$ and
$\{$
$\}$ and
$\{$
 $,$
$,$
 $,$
$,$
 $\}$
$\}$
 $,$
$,$
 $,$
$,$
 $\}$ and
$\{$
$\}$ and
$\{$
 $,$
$,$
 $,$
$,$
 $\}$
$\}$
Definition
Two events $A$ and $B$ are mutually exclusive exclusive if $$P(A \cap B)=0.$$
Example
Suppose you roll a die. The events $A$="roll an even number" and $B$="roll an odd number" cannot occur simultaneously. To see this,
$A=\{$
 $,$
$,$
 $,$
$,$
 $\}$ and
$B=\{$
$\}$ and
$B=\{$
 $,$
$,$
 $,$
$,$
 $\}$
$\}$
Thus $A \cap B =\varnothing$, so that $$P(A \cap B)=P(\varnothing)=0.$$
 $,$
$,$
 $,$
$,$
 $\}$ and
$B=\{$
$\}$ and
$B=\{$
 $,$
$,$
 $,$
$,$
 $\}$
$\}$
Addition Rule #1: If $A$ and $B$ are mutually exclusive events, then $$P(A \cup B)=P(A)+P(B).$$
Example
Draw a card from a standard, well-shuffled $52$-card deck.
What is the probability of drawing a queen or an ace?
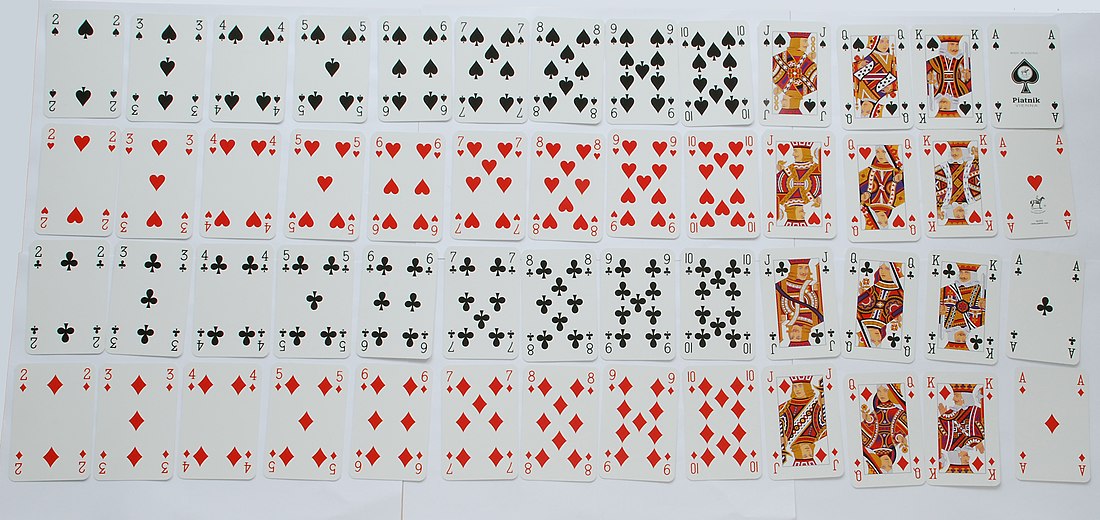
Let $Q$ be the event of "drawing a queen" and $A$ be the event "draw an ace."
Then the the event "drawing a queen or an ace" can be denoted as $Q\cup A.$
Now, we could represent these events as $Q=\{Q\diamondsuit,Q\heartsuit,Q\spadesuit,Q\clubsuit\}$ and $A=\{A\diamondsuit,A\heartsuit,A\spadesuit,A\clubsuit\}.$
Consequently, we see that $Q$ and $A$ are mutually exclusive.
We also see that $\displaystyle P(Q)=\frac{4}{52}=\frac{1}{13}$ and $\displaystyle P(A)=\frac{4}{52}=\frac{1}{13},$
With this information, we may now compute $P(Q \cup A).$ $$ \begin{array}{lll} \displaystyle P(Q \cup A)&\displaystyle=P(Q)+P(A) &\mbox{by Addition Rule #1}\\ \displaystyle &\displaystyle=\frac{1}{13}+\frac{1}{13} &\mbox{since $\displaystyle P(Q)=\frac{1}{13}$ and $\displaystyle P(A)=\frac{1}{13}$}\\ \displaystyle &\displaystyle=\frac{2}{13} &\mbox{}\\ \displaystyle &\displaystyle\approx 0.1538461538 &\mbox{}\\ \end{array} $$ We interpret this to mean that when we draw a card from a well-shuffled, $52$-card deck, about $15.4\%$ if the time, the card will be either an queen or an ace.
Then the the event "drawing a queen or an ace" can be denoted as $Q\cup A.$
Now, we could represent these events as $Q=\{Q\diamondsuit,Q\heartsuit,Q\spadesuit,Q\clubsuit\}$ and $A=\{A\diamondsuit,A\heartsuit,A\spadesuit,A\clubsuit\}.$
Consequently, we see that $Q$ and $A$ are mutually exclusive.
We also see that $\displaystyle P(Q)=\frac{4}{52}=\frac{1}{13}$ and $\displaystyle P(A)=\frac{4}{52}=\frac{1}{13},$
With this information, we may now compute $P(Q \cup A).$ $$ \begin{array}{lll} \displaystyle P(Q \cup A)&\displaystyle=P(Q)+P(A) &\mbox{by Addition Rule #1}\\ \displaystyle &\displaystyle=\frac{1}{13}+\frac{1}{13} &\mbox{since $\displaystyle P(Q)=\frac{1}{13}$ and $\displaystyle P(A)=\frac{1}{13}$}\\ \displaystyle &\displaystyle=\frac{2}{13} &\mbox{}\\ \displaystyle &\displaystyle\approx 0.1538461538 &\mbox{}\\ \end{array} $$ We interpret this to mean that when we draw a card from a well-shuffled, $52$-card deck, about $15.4\%$ if the time, the card will be either an queen or an ace.
Example
A pack of m&ms contains $\color{brown}{14}$ browns, $\color{#dbcc27}{10}$ yellows, $\color{green}{10}$ greens, $\color{red}{12}$ reds, $\color{darkorange}{12}$ oranges, and $\color{blue}{14}$ blues.
You draw one m&m from the bag.
Find the probability that you draw a green or a red m&m.
Let $G$ be the event "draw green" and $R$ be the event "draw red."
Now, $$ P(G)=\frac{\mbox{# ways $G$ can occur}}{\mbox{# of possible outcomes}}=\frac{\color{green}{10}}{\color{brown}{14}+\color{#dbcc27}{10}+\color{green}{10}+\color{red}{12}+\color{darkorange}{12}+\color{blue}{14}}=\frac{10}{72}=\frac{5}{36} $$ and $$ P(R)=\frac{\mbox{# ways $R$ can occur}}{\mbox{# of possible outcomes}}=\frac{\color{red}{12}}{\color{brown}{14}+\color{#dbcc27}{10}+\color{green}{10}+\color{red}{12}+\color{darkorange}{12}+\color{blue}{14}}=\frac{12}{72}=\frac{1}{6} $$ So, to "draw a green or red" is denoted by $G\cup R.$
Since these events cannot occur simultaneously, they are mutually exclusive.
So, $$ \begin{array}{lll} \displaystyle P(G\cup R)&\displaystyle=P(G)+P(R) &\mbox{by Addition Rule #1}\\ \displaystyle &\displaystyle=\frac{5}{36}+\frac{1}{6} &\mbox{}\\ \displaystyle &\displaystyle=\frac{5}{36}+\frac{6}{36} &\mbox{}\\ \displaystyle &\displaystyle=\frac{11}{36} &\mbox{}\\ \displaystyle &\displaystyle \approx 0.3055555556 &\mbox{}\\ \end{array} $$ Interpretation: Under the same conditions, this event would occur about $30.6\%$ of the time.
Now, $$ P(G)=\frac{\mbox{# ways $G$ can occur}}{\mbox{# of possible outcomes}}=\frac{\color{green}{10}}{\color{brown}{14}+\color{#dbcc27}{10}+\color{green}{10}+\color{red}{12}+\color{darkorange}{12}+\color{blue}{14}}=\frac{10}{72}=\frac{5}{36} $$ and $$ P(R)=\frac{\mbox{# ways $R$ can occur}}{\mbox{# of possible outcomes}}=\frac{\color{red}{12}}{\color{brown}{14}+\color{#dbcc27}{10}+\color{green}{10}+\color{red}{12}+\color{darkorange}{12}+\color{blue}{14}}=\frac{12}{72}=\frac{1}{6} $$ So, to "draw a green or red" is denoted by $G\cup R.$
Since these events cannot occur simultaneously, they are mutually exclusive.
So, $$ \begin{array}{lll} \displaystyle P(G\cup R)&\displaystyle=P(G)+P(R) &\mbox{by Addition Rule #1}\\ \displaystyle &\displaystyle=\frac{5}{36}+\frac{1}{6} &\mbox{}\\ \displaystyle &\displaystyle=\frac{5}{36}+\frac{6}{36} &\mbox{}\\ \displaystyle &\displaystyle=\frac{11}{36} &\mbox{}\\ \displaystyle &\displaystyle \approx 0.3055555556 &\mbox{}\\ \end{array} $$ Interpretation: Under the same conditions, this event would occur about $30.6\%$ of the time.
Big Question: What if two events are not mutually exclusive?!
Big Answer: Addition Rule #2
For any two events $A$ and $B,$ $$P(A \cup B)=P(A)+P(B)-P(A \cap B)$$
Example
What is the probability of drawing a red card or an odd-numbered card from a standard, well-shuffled $52$-card deck?

Let $R$ br the event "draw a red card" and $\mathscr{O}$ be the event "draw an odd-numbered card."
$$ R=\{A\diamondsuit,2\diamondsuit,3\diamondsuit,4\diamondsuit,5\diamondsuit,6\diamondsuit,7\diamondsuit,8\diamondsuit,9\diamondsuit,10\diamondsuit,J\diamondsuit,Q\diamondsuit,K\diamondsuit,A\heartsuit,2\heartsuit,3\heartsuit,4\heartsuit,5\heartsuit,6\heartsuit,7\heartsuit,8\heartsuit,9\heartsuit,10\heartsuit,J\heartsuit,Q\heartsuit,K\heartsuit\} $$ $$ \mathscr{O}=\{ 3\diamondsuit,5\diamondsuit,7\diamondsuit,9\diamondsuit,3\heartsuit,5\heartsuit,7\heartsuit,9\heartsuit,3\spadesuit,5\spadesuit,7\spadesuit,9\spadesuit,3\clubsuit,5\clubsuit,7\clubsuit,9\clubsuit \} $$ Then, $$ R\cap\mathscr{O}=\{ 3\diamondsuit,5\diamondsuit,7\diamondsuit,9\diamondsuit,3\heartsuit,5\heartsuit,7\heartsuit,9\heartsuit \} $$
From the above, we know that $\displaystyle P(R)=\frac{26}{52}=\frac{1}{2},$ $\displaystyle P(\mathscr{O})=\frac{16}{52}=\frac{4}{13},$ and $\displaystyle P(R\cap\mathscr{O})=\frac{8}{52}=\frac{2}{13},$
We may now compute the desired probability. $$ \begin{array}{lll} \displaystyle P(R\cup\mathscr{O})&\displaystyle= P(R)+P(\mathscr{O})-P(R\cap\mathscr{O})&\mbox{by Addition Rule #2}\\ \displaystyle &\displaystyle=\frac{26}{52}+\frac{16}{52}-\frac{8}{52} &\mbox{by the above}\\ \displaystyle &\displaystyle=\frac{34}{52} &\mbox{}\\ \displaystyle &\displaystyle=\frac{17}{26} &\mbox{}\\ \displaystyle &\displaystyle\approx 0.6538461538 &\mbox{}\\ \end{array} $$ We interpret this to mean that when we draw a card from a well-shuffled, $52$-card deck, about $65.4\%$ if the time, the card will be either an red card or an odd card.
$$ R=\{A\diamondsuit,2\diamondsuit,3\diamondsuit,4\diamondsuit,5\diamondsuit,6\diamondsuit,7\diamondsuit,8\diamondsuit,9\diamondsuit,10\diamondsuit,J\diamondsuit,Q\diamondsuit,K\diamondsuit,A\heartsuit,2\heartsuit,3\heartsuit,4\heartsuit,5\heartsuit,6\heartsuit,7\heartsuit,8\heartsuit,9\heartsuit,10\heartsuit,J\heartsuit,Q\heartsuit,K\heartsuit\} $$ $$ \mathscr{O}=\{ 3\diamondsuit,5\diamondsuit,7\diamondsuit,9\diamondsuit,3\heartsuit,5\heartsuit,7\heartsuit,9\heartsuit,3\spadesuit,5\spadesuit,7\spadesuit,9\spadesuit,3\clubsuit,5\clubsuit,7\clubsuit,9\clubsuit \} $$ Then, $$ R\cap\mathscr{O}=\{ 3\diamondsuit,5\diamondsuit,7\diamondsuit,9\diamondsuit,3\heartsuit,5\heartsuit,7\heartsuit,9\heartsuit \} $$
From the above, we know that $\displaystyle P(R)=\frac{26}{52}=\frac{1}{2},$ $\displaystyle P(\mathscr{O})=\frac{16}{52}=\frac{4}{13},$ and $\displaystyle P(R\cap\mathscr{O})=\frac{8}{52}=\frac{2}{13},$
We may now compute the desired probability. $$ \begin{array}{lll} \displaystyle P(R\cup\mathscr{O})&\displaystyle= P(R)+P(\mathscr{O})-P(R\cap\mathscr{O})&\mbox{by Addition Rule #2}\\ \displaystyle &\displaystyle=\frac{26}{52}+\frac{16}{52}-\frac{8}{52} &\mbox{by the above}\\ \displaystyle &\displaystyle=\frac{34}{52} &\mbox{}\\ \displaystyle &\displaystyle=\frac{17}{26} &\mbox{}\\ \displaystyle &\displaystyle\approx 0.6538461538 &\mbox{}\\ \end{array} $$ We interpret this to mean that when we draw a card from a well-shuffled, $52$-card deck, about $65.4\%$ if the time, the card will be either an red card or an odd card.
Example
In a Math $105$ class at SWOCC, there are $21$ first-year students and $12$ second-year students.
$5$ of the second-year students are female, and $11$ of the first-year students are male.
If we choose a random student from this class, what is the probability the student is a first-year student or female?
We shall organize the given information into a table.
$$
\begin{array}{|c|c|c|c|}
\hline
& \mbox{First Year} & \mbox{Second Year} & \mbox{Row Total}\\\hline
\mbox{Female} & & 5 & \\ \hline
\mbox{Male} & 11 & & \\ \hline
\mbox{Column Total} & 21 & 12 & \\ \hline
\end{array}
$$
Filling in the missing values,
$$
\begin{array}{|c|c|c|c|}
\hline
& \mbox{First Year} & \mbox{Second Year} & \mbox{Row Total}\\\hline
\mbox{Female} &\color{magenta}{10} & 5 & \color{magenta}{15}\\ \hline
\mbox{Male} & 11 & \color{magenta}{7} & \color{magenta}{18}\\ \hline
\mbox{Column Total} & 21 & 12 & \color{blue}{33}\\ \hline
\end{array}
$$
From our table we have
$$\displaystyle P(\mbox{First Year})=\frac{21}{33}$$
$$\displaystyle P(\mbox{Female})=\frac{15}{33}$$
$$\displaystyle P(\mbox{First Year}\cap \mbox{Female})=\frac{10}{33}$$
From these probabilities we may now compute the desired probability.
$$
\begin{array}{lll}
\displaystyle P(\mbox{First Year}\cup \mbox{Female})&\displaystyle=P(\mbox{First Year})+P(\mbox{Female})-P(\mbox{First Year}\cap \mbox{Female}) &\mbox{by Addition Rule #2}\\
\displaystyle &\displaystyle=\frac{21}{33}+\frac{15}{33}-\frac{10}{33} &\mbox{}\\
\displaystyle &\displaystyle=\frac{26}{33} &\mbox{}\\
\displaystyle &\displaystyle\approx 0.7878787879 &\mbox{}\\
\end{array}
$$
We interpret this to mean that if we choose a student from this class at random,
about $78.8\%$ of the time the chosen student will be either a first-year student or a female student.