We now develop techniques for finding derivatives of another class of transcendental functions.
Exponential and logarithmic functions are good at modelling growth.
A Very Fundamental Derivative: We shall begin by considering the derivative of the function $f(x)=e^x.$ Using the limit definition of the derivative, $$ \begin{array}{lll} \displaystyle \frac{d}{dx}e^x &\displaystyle=\lim_{h \rightarrow 0}\frac{f(x+h)-f(x)}{h}&\mbox{definition of the derivative}\\ &\displaystyle=\lim_{h \rightarrow 0}\frac{e^{x+h}-e^x}{h}&\mbox{}\\ &\displaystyle=\lim_{h \rightarrow 0}\frac{e^{x}e^{h}-e^x}{h}&\\ &\displaystyle=\lim_{h \rightarrow 0}\frac{(e^{h}-1)e^x}{h}&\\ &\displaystyle=\lim_{h \rightarrow 0}\frac{e^{h}-1}{h}e^x&\\ \end{array} $$ Thus, finding $\displaystyle \frac{d}{dx}e^x$ amounts to evaluating the limit $\displaystyle \lim_{h \rightarrow 0}\frac{e^{h}-1}{h}.$
A Very Fundamental Derivative $$\lim_{h \rightarrow 0}\frac{e^{h}-1}{h}$$
| $h$ | $\displaystyle \frac{e^h-1}{h}$ |
A Very Fundamental Derivative
$$\frac{d}{dx} e^x = e^x$$
Example
$$\frac{d}{dx} 3^x$$
$$
\begin{array}{lll}
\displaystyle \frac{d}{dx}3^x &\displaystyle=\displaystyle \frac{d}{dx}e^{\ln(3^x)}& \mbox{$e^x$ and $\ln x$ are inverses}\\
&=\displaystyle \frac{d}{dx}e^{x\ln 3}&\mbox{Logs bring exponents down to earth.}\\
&=\displaystyle e^{x\ln 3}\frac{d}{dx}(x\ln 3)&\mbox{by the Chain Rule}\\
&=\displaystyle e^{x\ln(3)}(\ln 3)&\mbox{$\frac{d}{dx}(cx)=c$}\\
&=\displaystyle e^{\ln(3^x)}(\ln 3)&\mbox{Logs can put exponents back on top.}\\
&=\displaystyle 3^x (\ln 3)&\mbox{$e^x$ and $\ln x$ are inverses}\\
\end{array}
$$
Derivatives of Exponential Functions
$$\frac{d}{dx} b^x =b^x \ln b$$
Dire Warning
WHEN YOU DO THIS
$\displaystyle \frac{d}{dx}3^x=x3^{x-1}$
YOU MAKE KITTY ANGRY!!!!!!!!!!!!!!!!!!


Another Fundamental Derivative
Suppose we want to find the derivative of $y=\ln x.$
We can pull the same trick (AGAIN!) we did with generic inverse functions by rearranging by writing $$x=e^{\ln x}$$ and then differentiating both sides using the chain rule.
$$
\begin{array}{lll}
&x=e^{\ln x}&\\
\implies & \displaystyle \frac{d}{dx}x=\frac{d}{dx}e^{\ln x}&\mbox{differentiate both sides with respect to $x$}\\
\implies & \displaystyle 1=e^{\ln x}\frac{d}{dx}\ln x&\mbox{by the Chain Rule}\\
\implies & \displaystyle 1=x\frac{d}{dx}\ln x&\mbox{$e^x$ and $\ln x$ are inverses}\\
\implies & \displaystyle \frac{1}{x}=\frac{d}{dx} \ln x&\mbox{}\\
\end{array}
$$
Another Fundamental Derivative
$$\frac{d}{dx}\ln x=\frac{1}{x}$$
Example
$$\frac{d}{dx} \log_3 x$$
$$
\begin{array}{lll}
\displaystyle \frac{d}{dx}\log_3 x &\displaystyle=\displaystyle \frac{d}{dx}\frac{\ln x}{\ln 3}& \mbox{by the change of base formula}\\
&=\displaystyle \frac{d}{dx}\left(\frac{1}{\ln 3}\ln x\right)&\mbox{}\\
&=\displaystyle \frac{1}{\ln 3} \frac{d}{dx}\ln x&\mbox{$\frac{1}{\ln 3}$ is just a constant we can pull out}\\
&=\displaystyle \frac{1}{\ln 3} \frac{1}{x}&\mbox{ $\frac{d}{dx}\ln x=\frac{1}{x}$}\\
&=\displaystyle \frac{1}{x\ln 3}&\mbox{}\\
\end{array}
$$
Derivatives of Logarithmic Functions
$$\frac{d}{dx} \log_b x =\frac{1}{x \ln b}$$
Example
Find $f'(x)$ for the following.
$\displaystyle f(x)=\sqrt{e^{2x}+2x}$
$$
\begin{array}{lll}
\displaystyle f'(x) &=\displaystyle \frac{d}{dx}\sqrt{e^{2x}+2x}& \mbox{}\\
&=\displaystyle \frac{d}{dx}(e^{2x}+2x)^{1/2}& \mbox{}\\
&=\displaystyle \frac{1}{2}(e^{2x}+2x)^{-1/2}\frac{d}{dx}(e^{2x}+2x)& \mbox{}\\
&=\displaystyle \frac{1}{2}(e^{2x}+2x)^{-1/2}(2e^{2x}+2)& \mbox{$\frac{d}{dx}e^{2x}=e^{2x}\frac{d}{dx}(2x)=2e^{2x}$}\\
&=\displaystyle \frac{1}{2}(e^{2x}+2x)^{-1/2}\cdot 2(e^{2x}+1)& \mbox{factor out 2}\\
&=\displaystyle (e^{2x}+2x)^{-1/2}(e^{2x}+1)& \mbox{cancel out 2}\\
&=\displaystyle \frac{1}{\sqrt{e^{2x}+2x}}(e^{2x}+1)& \mbox{get into radical form}\\
&=\displaystyle \frac{e^{2x}+1}{\sqrt{e^{2x}+2x}}& \mbox{put on finishing touches}\\
\end{array}
$$
Example
$\displaystyle f(x)=3^{\sin 3x}$
$$
\begin{array}{lll}
f'(x) &=\displaystyle \frac{d}{dx} 3^{\sin 3x}& \mbox{}\\
&=\displaystyle 3^{\sin 3x}\ln 3 \frac{d}{dx} \sin 3x& \mbox{by the Chain Rule}\\
&=\displaystyle 3^{\sin 3x}\ln 3 \cos 3x \frac{d}{dx}3x& \mbox{by the Chain Rule}\\
&=\displaystyle 3^{\sin 3x}\ln 3 \cos 3x \cdot 3& \mbox{}\\
&=\displaystyle 3 \ln 3 \cdot 3^{\sin 3x} \cos 3x & \mbox{}\\
&=\displaystyle \ln 3^3 \cdot 3^{\sin 3x} \cos 3x & \mbox{}\\
&=\displaystyle \ln 27 \cdot 3^{\sin 3x} \cos 3x & \mbox{}\\
\end{array}
$$
Example
$\displaystyle f(x)=\ln \sqrt{5x-7}$
Version 1:
$$
\begin{array}{lll}
f'(x) &=\displaystyle \frac{d}{dx} \ln \sqrt{5x-7}& \mbox{}\\
&=\displaystyle \frac{1}{\sqrt{5x-7}}\frac{d}{dx}\sqrt{5x-7}& \mbox{by the Chain Rule}\\
&=\displaystyle \frac{1}{\sqrt{5x-7}}\frac{d}{dx}(5x-7)^{1/2}& \mbox{}\\
&=\displaystyle \frac{1}{\sqrt{5x-7}}\frac{1}{2}(5x-7)^{-1/2}\frac{d}{dx}(5x-7)& \mbox{by the Chain Rule}\\
&=\displaystyle \frac{1}{\sqrt{5x-7}}\frac{1}{2\sqrt{5x-7}}\cdot 5& \mbox{}\\
&=\displaystyle \frac{5}{2(5x-7)}& \mbox{}\\
&=\displaystyle \frac{5}{10x-14}& \mbox{}\\
\end{array}
$$
Version 2: Use properties of logs.
$$
\begin{array}{lll}
f'(x) &=\displaystyle \frac{d}{dx} \ln \sqrt{5x-7}& \mbox{}\\
&=\displaystyle \frac{d}{dx} \ln (5x-7)^{1/2}& \mbox{}\\
&=\displaystyle \frac{d}{dx} \left(\frac{1}{2}\ln (5x-7)\right)& \mbox{}\\
&=\displaystyle \frac{1}{2}\frac{d}{dx}\ln (5x-7)& \mbox{}\\
&=\displaystyle \frac{1}{2}\frac{1}{5x-7}\frac{d}{dx}(5x-7)& \mbox{by the Chain Rule}\\
&=\displaystyle \frac{1}{2(5x-7)}\cdot 5& \mbox{}\\
&=\displaystyle \frac{5}{10x-14}& \mbox{}\\
\end{array}
$$
Example
$\displaystyle f(x)=\log_7 \left(6x^4+3\right)^5$
$$
\begin{array}{lll}
f'(x) &=\displaystyle \frac{d}{dx} \log_7 \left(6x^4+3\right)^5& \mbox{}\\
&=\displaystyle \frac{d}{dx} \left(5\log_7\left(6x^4+3\right)\right)& \mbox{}\\
&=\displaystyle 5 \frac{d}{dx} \log_7\left(6x^4+3\right)& \mbox{}\\
&=\displaystyle 5 \frac{1}{(6x^4+3)\ln 7}\frac{d}{dx}(6x^4+3)& \mbox{by the Chain Rule}\\
&=\displaystyle \frac{5}{(6x^4+3)\ln 7}(24x^3)& \mbox{}\\
&=\displaystyle \frac{120x^3}{(6x^4+3)\ln 7}& \mbox{}\\
&=\displaystyle \frac{40x^3}{(2x^4+1)\ln 7}& \mbox{}\\
\end{array}
$$
Weird Example
$$\frac{d}{dx} x^x$$ Any ideas?
$$
\begin{array}{lll}
&y=x^x&\\
\implies &\ln y=\ln x^x&\\
\implies &\ln y=x\ln x&\mbox{Logs bring exponents down to earth!}\\
\implies & \displaystyle \frac{d}{dx}\ln y=\frac{d}{dx}(x \ln x)&\mbox{differentiate both sides with respect to $x$}\\
\implies & \displaystyle \frac{1}{y}\frac{dy}{dx}=(x)'(\ln x)+(x)(\ln x)'&\mbox{by the Chain Rule (left) the Product Rule (right)}\\
\implies & \displaystyle \frac{1}{y}\frac{dy}{dx}=\ln x+x\frac{1}{x}&\mbox{}\\
\implies & \displaystyle \frac{1}{y}\frac{dy}{dx}=\ln x+1&\mbox{}\\
\implies & \displaystyle \frac{dy}{dx}=y(\ln x+1)&\mbox{}\\
\implies & \displaystyle \frac{dy}{dx}=x^x(\ln x+1)&\mbox{since $y=x^x$}\\
\end{array}
$$
Logarithmic Differentiation
1. To differentiate $y=h(x)$ using logarithmic differentiation, take the natural logarithm of both sides of the equation to obtain $\ln y= \ln(h(x)).$
2. Use properties of logarithms to expand $\ln(h(x))$ as much as possible.
3. Implicitly differentiate both sides of the equation. On the left we will have $\displaystyle \frac{1}{y}\frac{dy}{dx}.$
4. Multiply both sides of the equation by $y$ to solve for $\displaystyle \frac{dy}{dx}.$
5. Replace $y$ by $h(x).$
Product/Quotient-Rule-Heavy Examples
Use logarithmic differentiation to find the derivative of $$y=\frac{x+11}{\sqrt[3]{x^2-4}}.$$
$$
\begin{array}{lll}
&\displaystyle y=\frac{x+11}{\sqrt[3]{x^2-4}}&\\
\implies &\displaystyle \ln y=\displaystyle \ln \frac{x+11}{\sqrt[3]{x^2-4}}& \mbox{Take a natural log of both sides.}\\
\implies &\displaystyle \ln y=\displaystyle \ln (x+11)-\ln(\sqrt[3]{x^2-4})&\mbox{A log of quotient is a difference of the logs.}\\
\implies &\displaystyle \ln y=\displaystyle \ln (x+11)-\ln((x^2-4)^{1/3})&\mbox{}\\
\implies &\displaystyle \ln y=\displaystyle \ln (x+11)-\frac{1}{3}\ln(x^2-4)&\mbox{Logs bring exponents down to earth.}\\
\implies &\displaystyle \frac{d}{dx} \ln y=\displaystyle \frac{d}{dx} \left(\ln (x+11)-\frac{1}{3}\ln(x^2-4)\right)&\mbox{Differentiate both sides.}\\
\implies &\displaystyle \frac{1}{y}\frac{dy}{dx}=\displaystyle \frac{d}{dx}\ln (x+11)-\frac{1}{3}\frac{d}{dx}\ln(x^2-4)&\mbox{by the Chain Rule (left)}\\
\implies &\displaystyle \frac{1}{y}\frac{dy}{dx}=\displaystyle \frac{1}{x+11}\frac{d}{dx}(x+11)-\frac{1}{3}\frac{1}{x^2-4}\frac{d}{dx}(x^2-4)&\mbox{by the Chain Rule (right)}\\
\implies &\displaystyle \frac{1}{y}\frac{dy}{dx}=\displaystyle \frac{1}{x+11}-\frac{1}{3}\frac{1}{x^2-4}(2x)&\mbox{}\\
\implies &\displaystyle \frac{1}{y}\frac{dy}{dx}=\displaystyle \frac{1}{x+11}-\frac{2x}{3(x^2-4)}&\mbox{}\\
\implies &\displaystyle \frac{dy}{dx}=\displaystyle y\left(\frac{1}{x+11}-\frac{2x}{3(x^2-4)}\right)&\mbox{}\\
\implies &\displaystyle \frac{dy}{dx}=\displaystyle \frac{x+11}{\sqrt[3]{x^2-4}} \left(\frac{1}{x+11}-\frac{2x}{3(x^2-4)}\right)&\mbox{since $y=\frac{x+11}{\sqrt[3]{x^2-4}}$}\\
\end{array}
$$
If required, we may need to clean up our answer a bit:
$$
\begin{array}{lll}
\displaystyle \frac{dy}{dx}&=\displaystyle \frac{x+11}{\sqrt[3]{x^2-4}} \left(\frac{1}{x+11}-\frac{2x}{3(x^2-4)}\right)&\\
&=\displaystyle \frac{x+11}{\sqrt[3]{x^2-4}}\frac{1}{x+11}-\frac{x+11}{\sqrt[3]{x^2-4}}\frac{2x}{3(x^2-4)}&\mbox{distribute}\\
&=\displaystyle \frac{1}{{\sqrt[3]{x^2-4}}}-\frac{x+11}{\sqrt[3]{x^2-4}}\frac{2x}{3(x^2-4)}&\mbox{cancel $x+11$}\\
&=\displaystyle \frac{1}{{\sqrt[3]{x^2-4}}}-\frac{2x(x+11)}{3(x^2-4)^{4/3}}&\mbox{multiply fractions}\\
&=\displaystyle \frac{1}{{\sqrt[3]{x^2-4}}}\frac{3(x^2-4)}{3(x^2-4)}-\frac{2x(x+11)}{3(x^2-4)^{4/3}}&\mbox{multiply by fancy one to get common denominator}\\
&=\displaystyle \frac{3(x^2-4)}{3(x^2-4)^{4/3}}-\frac{2x(x+11)}{3(x^2-4)^{4/3}}&\mbox{}\\
&=\displaystyle \frac{3x^2-12}{3(x^2-4)^{4/3}}-\frac{2x^2+22x}{3(x^2-4)^{4/3}}&\mbox{expand numerators}\\
&=\displaystyle \frac{3x^2-12-2x^2-22x}{3(x^2-4)^{4/3}}&\mbox{subtract (don't forget to distribut minus!)}\\
&=\displaystyle \frac{x^2-22x-12}{3(x^2-4)^{4/3}}&\mbox{simplify}\\
&=\displaystyle \frac{x^2-22x-12}{3\sqrt[3]{(x^2-4)^4}}&\mbox{express in radical notation if desired}\\
\end{array}
$$
Application
The graph below is an exponential fit of the population of my hometown where $t$ is the number of years since $1860.$ The red function $P(t)$ is given by $$P(t) = 1318.3462 \cdot (1.008)^t .$$ Use the function $P$ to estimate the rate at which the population is growing in the year $2020.$
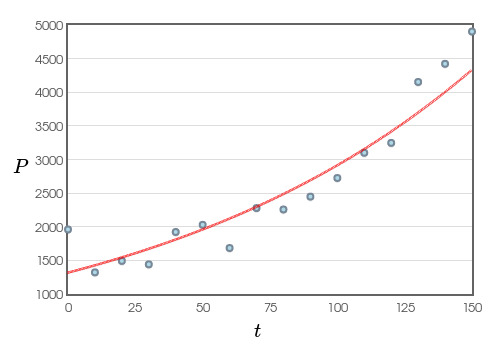
Since time $t$ is measured in years since $1860,$ we need to find $P'(160).$ First, however, we must find $P'(t):$
$$
\begin{array}{lll}
P'(t) &=\displaystyle \frac{d}{dt} (1318.3462 \cdot (1.008)^t)& \mbox{}\\
&=\displaystyle 1318.3462 \frac{d}{dt} 1.008^t& \mbox{}\\
&=\displaystyle 1318.3462 \cdot 1.008^t \cdot (\ln 1.008)& \mbox{}\\
&=\displaystyle 1318.3462 \cdot \ln 1.008 \cdot 1.008^t & \mbox{}\\
&\approx \displaystyle 10.5048 \cdot 1.008^t & \mbox{}\\
\end{array}
$$
We may now estimate $P'(160):$
$$
\begin{array}{lll}
P'(160) &\approx \displaystyle 10.5048 \cdot 1.008^{160} & \mbox{}\\
&\approx 37.5901 & \mbox{}\\
\end{array}
$$
That is, in $2020,$ we estimate that the population was growing by about $38$ people per year.