Today we apply our ever-growing knowledge of how interest works to understanding a type of loan many of us likely will, or have already encountered: mortgages.
Mortgages
Most people who are interested in purchasing a home will need to take out a loan.
Home loans are most typically mortgages.
A mortgage is a type of loan that is guaranteed by the very thing being purchased by the borrower. If a borrower defaults on the loan, the lender has the right to seize the purchased item be it real estate, a car, etc.

Mortgages
The term of a mortgage is typically $30$ years. This is because payments are lower.
On the other hand, it is possible to secure mortgages with shorter terms, for example $15$ years is fairly common, but the payments are higher.
As we shall see, despite the lower payments on a $30$-year mortgage, the amount of interest paid over the life of the loan can be substantially higher than a shorter-term loan.

Mortgages
Most mortgages are fixed-rate mortgages. That is, the interest rate doesn't change over the life of the loan.
On the other hand, some mortgages are adjustable-rate mortgages whose rate can vary from payment period to payment period.
Today, we will focus only on fixed-rate mortgages.

Fixed-Rate Mortgages and Payments
Like any loan, mortgages have a principal amount $P$ borrowed, and an annual interest rate $r.$
If $n$ payments are made per year, then the monthly payment $R$ is calculated as $$R=\frac{Pr/n}{1-\left( 1+r/n \right)^{-nt}}$$ Typically payments are monthly, in which case $n=12.$
Example
Mr. Holt is buying a house for $\$235000$ and has paid a down payment of $24\%.$ The remainder will be financed with a $15$-year mortgage at $6.6\%$ annual interest.
(a) What is the principal amount of the loan?
The principal amount of the loan is $\$235000$ minus $24\%.$
That is, $235000-0.24\cdot 235000=178600.$
So, the principal amount of the loan is $\$178600.$
That is, $235000-0.24\cdot 235000=178600.$
So, the principal amount of the loan is $\$178600.$
(b) Determine Mr. Holt's monthly payment.
The monthly payment is determined by the Fixed-Rate Mortgage formula with
$$
P=178600,\,\,\,r=0.066,\,\,\,n=12,\,\,\,\mbox{and}\,\,\,t=15
$$
Then
$$
\begin{array}{lll}
\displaystyle R&\displaystyle=\frac{Pr/n}{1-\left( 1+r/n \right)^{-nt}} &\mbox{}\\
\displaystyle &\displaystyle=\frac{178600\cdot 0.066/12}{1-\left( 1+0.066/12 \right)^{-12\cdot 15}} &\mbox{}\\
\displaystyle &\displaystyle=1565.632857 &\mbox{}\\
\end{array}
$$
The monthly payment is $\$1565.63.$
The calculator input will look something like $$ \mbox{(178600*0.066/12)/(1-(1+0.066/12)^(-12*15))} $$
The calculator input will look something like $$ \mbox{(178600*0.066/12)/(1-(1+0.066/12)^(-12*15))} $$
(c) How much will Mr. Holt be paying in interest over the life of the loan.
Mr. Holt will be making monthly payments of $\$1565.63$ for $15$ years.
That is, Mr. Holt will be making a total of $12\cdot 15=180$ payments of $\$1565.63.$
So, in total Mr. Holt will be paying back $180\cdot \$1565.63=\$281813.40.$
Thus, the total interest Mr. Holt will pay is this total amount paid minus the principal amount of the loan.
That is, $281813.40-178600=103213.4$
Mr. Holt will be paying $\$103213.4$ in interest over the life of the loan.
That is, Mr. Holt will be making a total of $12\cdot 15=180$ payments of $\$1565.63.$
So, in total Mr. Holt will be paying back $180\cdot \$1565.63=\$281813.40.$
Thus, the total interest Mr. Holt will pay is this total amount paid minus the principal amount of the loan.
That is, $281813.40-178600=103213.4$
Mr. Holt will be paying $\$103213.4$ in interest over the life of the loan.
(d) Suppose Mr. Holt took had taken out a $30$-year mortgage instead. What would his monthly payment have been?
The monthly payment is determined by the Fixed-Rate Mortgage formula with
$$
P=178600,\,\,\,r=0.066,\,\,\,n=12,\,\,\,\mbox{and}\,\,\,t=\color{magenta}{30}
$$
Then
$$
\begin{array}{lll}
\displaystyle R&\displaystyle=\frac{Pr/n}{1-\left( 1+r/n \right)^{-nt}} &\mbox{}\\
\displaystyle &\displaystyle=\frac{178600\cdot 0.066/12}{1-\left( 1+0.066/12 \right)^{-12\cdot \color{magenta}{30}}} &\mbox{}\\
\displaystyle &\displaystyle=1140.644649 &\mbox{}\\
\end{array}
$$
The monthly payment would have been $\$1140.64.$
The calculator input will look something like $$ \mbox{(178600*0.066/12)/(1-(1+0.066/12)^(-12*30))} $$
The calculator input will look something like $$ \mbox{(178600*0.066/12)/(1-(1+0.066/12)^(-12*30))} $$
(e) How much interest would he have paid over the life of the loan for the $30$-year term?
Mr. Holt would be making monthly payments of $\$1140.64$ for $30$ years.
That is, Mr. Holt would be making a total of $12\cdot 30=360$ payments of $\$1140.64.$
So, in total Mr. Holt would be paying back $360\cdot \$1140.64=\$410630.40.$
Thus, the total interest Mr. Holt would have paid is this total amount paid minus the principal amount of the loan.
That is, $410630.40-178600=232030.4$
Mr. Holt would have paid $\$232030.4$ in interest over the life of the loan.
That is, Mr. Holt would be making a total of $12\cdot 30=360$ payments of $\$1140.64.$
So, in total Mr. Holt would be paying back $360\cdot \$1140.64=\$410630.40.$
Thus, the total interest Mr. Holt would have paid is this total amount paid minus the principal amount of the loan.
That is, $410630.40-178600=232030.4$
Mr. Holt would have paid $\$232030.4$ in interest over the life of the loan.
Amortization Schedules
A lending institution will often prepare for borrowers an amortization schedule.
The amortization schedule tells the borrower how much of their monthly payment goes to interest and how much goes to the principal.
Each month, the balance on the principal is computed, and the monthly payment covers the interest.
The remainder of the payment goes to the principal balance.
Example
Mr. Holt's principal balance of $\$178600$ is being financed with a $15$-year mortgage at $6.6\%$ annual interest.
What will be the first two lines of the amortization schedule?
The after the first month, the interest is on the principal balance is
$$
I=Prt=178600\cdot 0.066\cdot \frac{1}{12}=982.30
$$
The monthly payment $\$1565.63$ pays this interest, and remainder $\$1565.63-\$982.30=\$583.33$
pays down the principal balance.
So, after the first payment is made, the principal balance is $\$178600-\$583.33=\$178016.67$
The first entry of the amortization schedule is
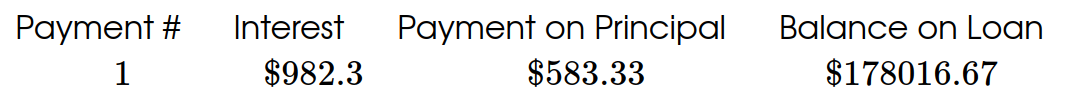
The after the second month, the interest is on the principal balance is $$ I=Prt=178016.67\cdot 0.066\cdot \frac{1}{12}=979.09 $$ The monthly payment $\$1565.63$ pays this interest, and remainder $\$1565.63-\$979.09=\$586.54$ pays down the principal balance.
So, after the second payment is made, the principal balance is $\$178016.67-\$586.54=\$177430.13$
The first two entries of the amortization schedule are

So, after the first payment is made, the principal balance is $\$178600-\$583.33=\$178016.67$
The first entry of the amortization schedule is
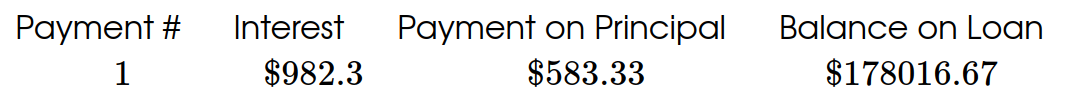
The after the second month, the interest is on the principal balance is $$ I=Prt=178016.67\cdot 0.066\cdot \frac{1}{12}=979.09 $$ The monthly payment $\$1565.63$ pays this interest, and remainder $\$1565.63-\$979.09=\$586.54$ pays down the principal balance.
So, after the second payment is made, the principal balance is $\$178016.67-\$586.54=\$177430.13$
The first two entries of the amortization schedule are

Example
In the previous example we calculated our monthly payment We will now calculate the first few lines of the amortization table.
| Payment # | Interest | Payment on Principal | Balance on Loan |